
Hippocrates(460-370 BC), known as the father of medicine, was a physician during the Pericles era in ancient Greece.

The Hippocratic school that bears his name separated medicine from magic and philosophy,
created the profession of doctor, and revolutionized ancient Greek medicine.
The records that remain are mixed up, so the details of his thoughts and actions are not known.

Nevertheless, Hippocrates is recorded as a typical ancient doctor, and he made numerous records while treating patients,
including organizing and implementing the ideas of previous schools.
He developed therapeutic medicine and created the Hippocratic Oath.


After numerous studies, he founded scientific medicine,
which established the medical principle that all diseases are caused by natural causes.
It also influenced the way of thinking of the Greeks at the time, creating a collection of papers called
“Hippocratic Medical Collection” written by numerous doctors and philosophers.

Hippocrates of the Crescent (470-410 BC) also played an important role in the history of mathematics.
When the enemy problem was a big issue in ancient Greece,
the relationship between the area of the crescent moon sandwiched between a right triangle within a quadrant
and an arc was discovered.
Now, shall we solve Hippocrates’ crescent moon problem?
“Identify curves with straight lines.”
It's not just absurd.
Hippocrates of Chios had already calculated the area of a curved area.
That is the Crescent of Hippocrates.
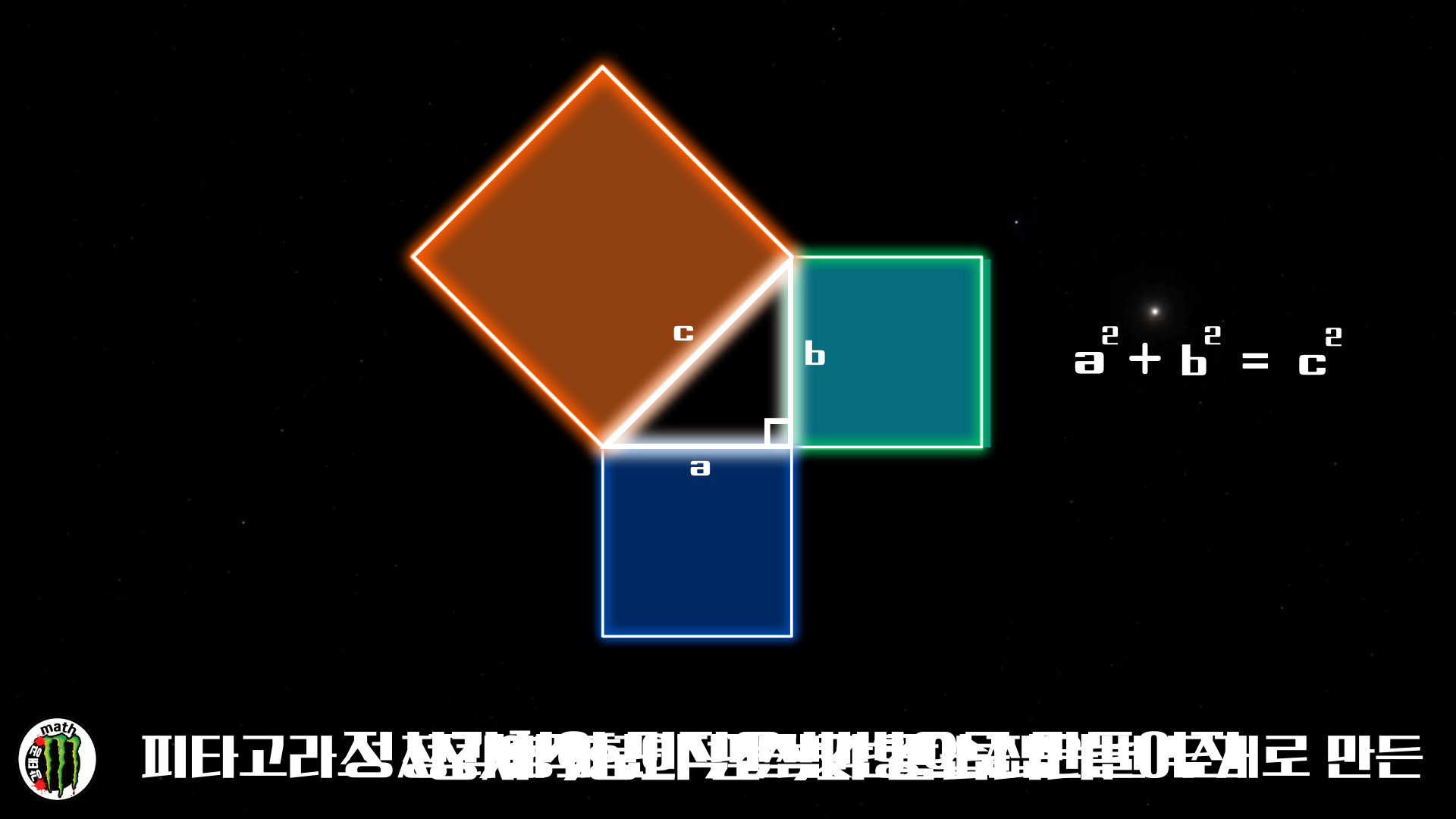
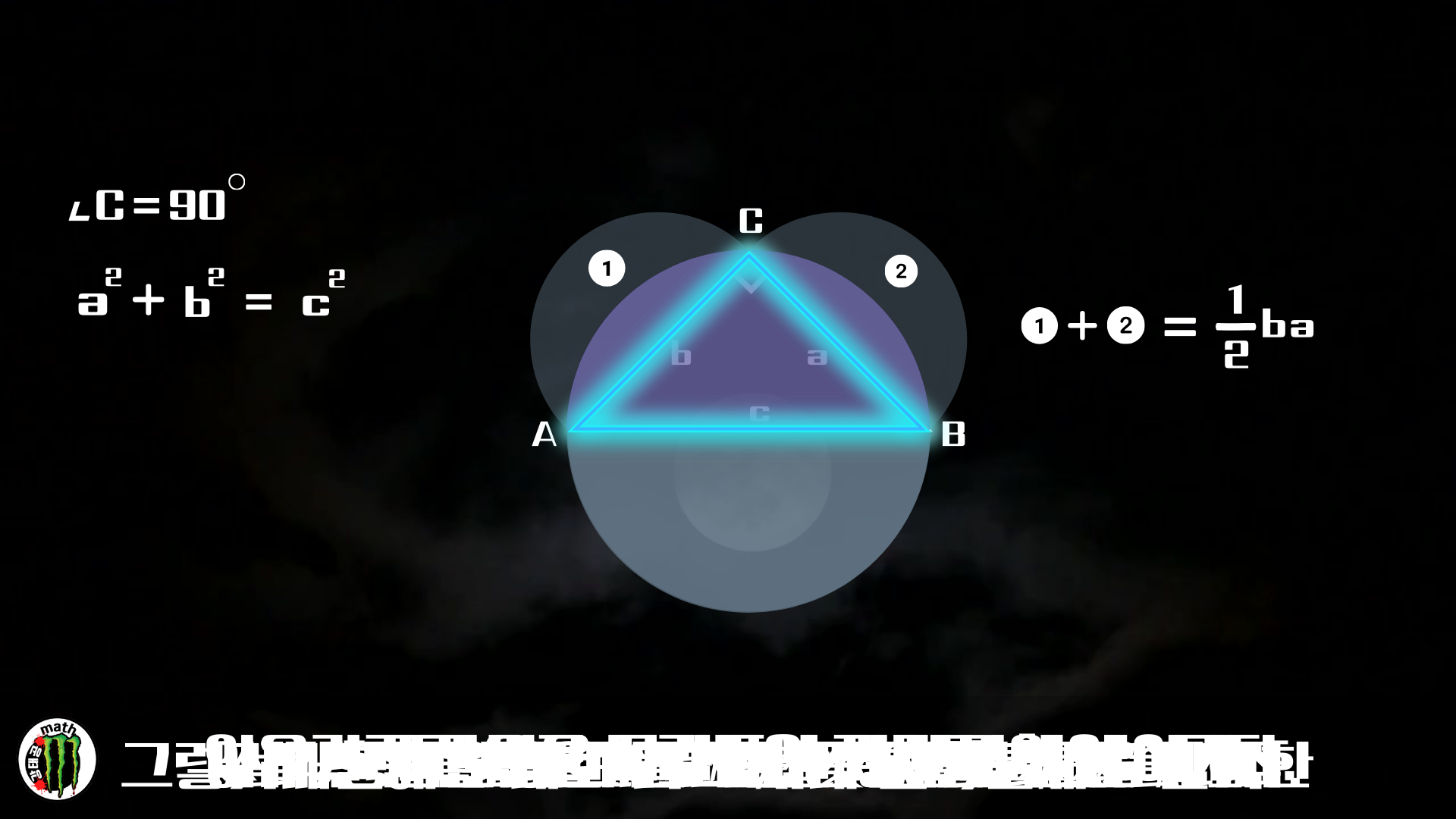
According to the Pythagorean theorem, the area of the square formed by the two short sides of a right triangle
is equal to the area of the square formed by the hypotenuse.

No matter how it changes, the two areas above are the same as the one below.
The same applies when you are a class member.
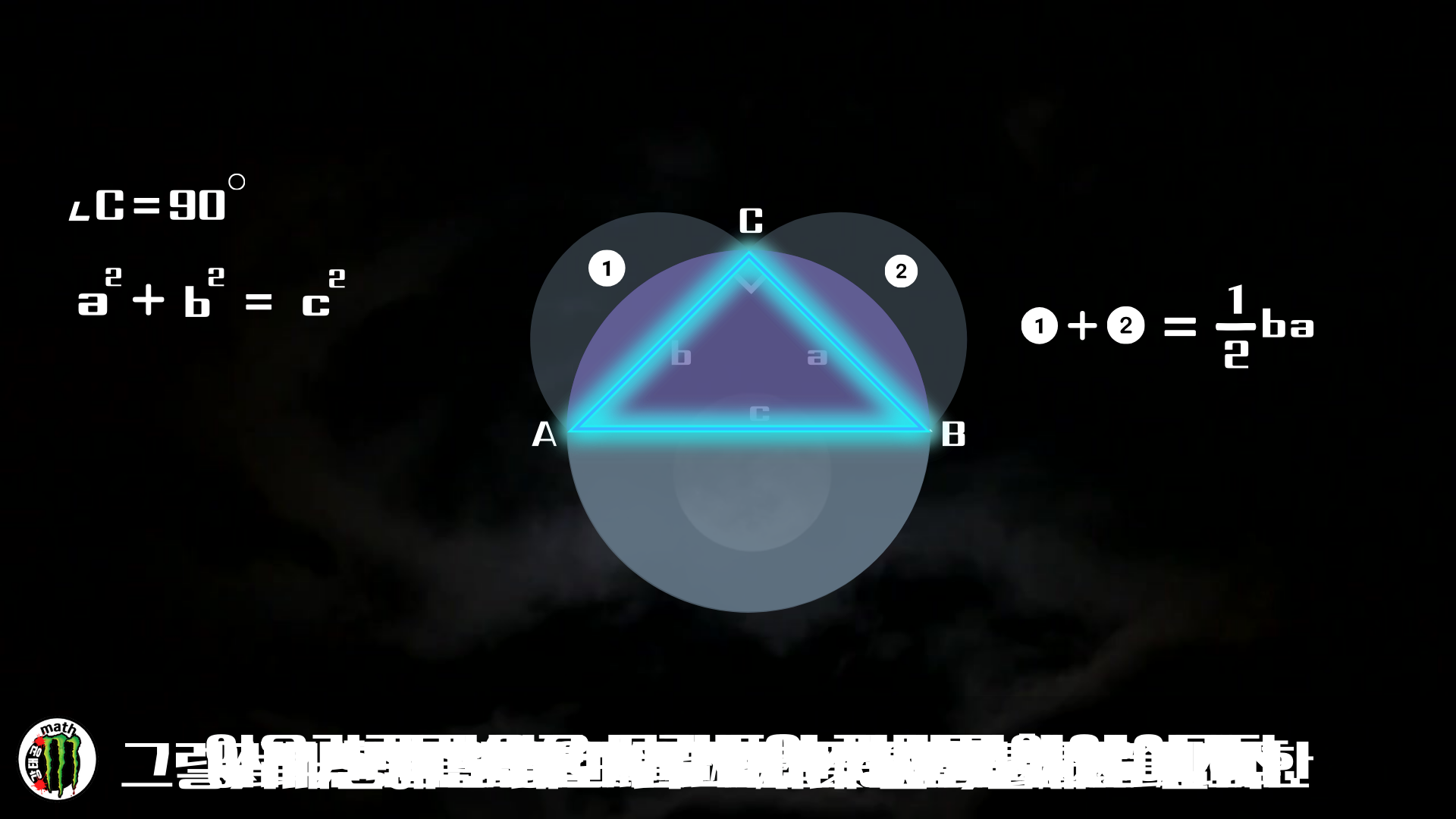
If you raise the lower semicircle up, you can see that the area of that part surrounded by the curve
excluding the common part is the same as the area of the triangle.
Therefore, many people expected that it would be possible to solve the problem of enemies during the ancient Greek era.

The problem covered in Shorts is also a problem that shows that the area of
the area surrounded by the curve is the same as the area of the triangle.
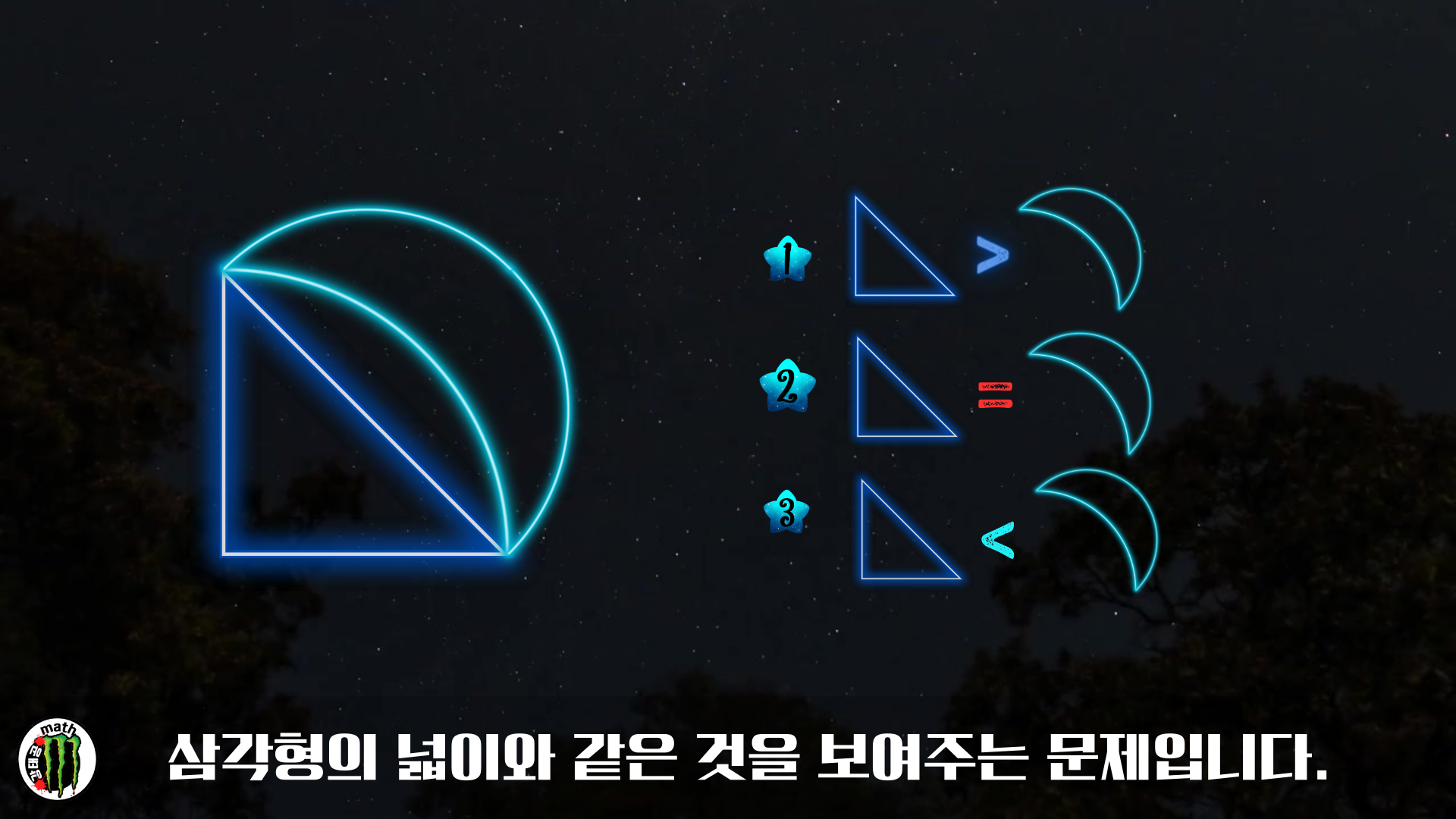
If the length of the short side of a right isosceles triangle is 2, we can see that the area of the quadrant is π.
And we can see that the area of a semicircle with the hypotenuse as its diameter is also π.

Since the areas of the two parts are the same,
we can see that the area of the right triangle and the area of the crescent shape are the same.
I was surprised to learn that the Hippocratic crescent moon,
which had been difficult in middle school, could be seen simply by folding it up.
This is ktgmath, a math knowledge channel. thank you
This is a YouTube video link.
https://youtu.be/nTMTrG50XDQ?si=ILh-yn74NEp4UnaG



